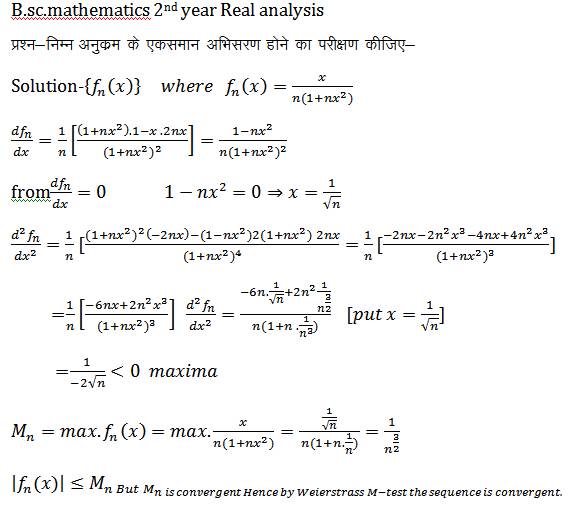
uniform convergence by mn test
श्रेणी के एकसमान अभिसरण के लिए एमएन परीक्षण (Uniform Convergence By mn test):
- श्रेणी के एकसमान अभिसरण के लिए एमएन परीक्षण (Uniform Convergence By mn test):
Uniform Convergence of Sequences:Let the sequence <f_{n}(x)> converge for every point x in I i.e.,the function f_{n} tends to limit as n\longrightarrow{\infin} for every x in I.This limit will be a function of x,say f.It follows from the definition of a limit that for every \epsilon there exits a positive integer m such that
|f_{n}(x)-f(x)|<\epsilon \text{ whenever }n\geq{m}
This integer m will depend upon x as well as \epsilon and so we can write it as m\left(x,\epsilon \right).Now if we keep \epsilon fixed and very x, then for a given point x in I there corresponds a value of m\left(x,\epsilon \right).Thus we shall find a set of values of m\left(x,\epsilon \right). This set may or may not have an upper bound.If this set has an upper bound say M, then for every point x in I,we get
|f_{n}(x)-f(x)|<\epsilon \text{ whenever }n\geq{m}
In this case the sequence <f_{n}> is said to converge uniformly to f on I. - आपको यह जानकारी रोचक व ज्ञानवर्धक लगे तो अपने मित्रों के साथ इस गणित के आर्टिकल को शेयर करें।यदि आप इस वेबसाइट पर पहली बार आए हैं तो वेबसाइट को फॉलो करें और ईमेल सब्सक्रिप्शन को भी फॉलो करें।जिससे नए आर्टिकल का नोटिफिकेशन आपको मिल सके । यदि आर्टिकल पसन्द आए तो अपने मित्रों के साथ शेयर और लाईक करें जिससे वे भी लाभ उठाए । आपकी कोई समस्या हो या कोई सुझाव देना चाहते हैं तो कमेंट करके बताएं।इस आर्टिकल को पूरा पढ़ें।
(If you find this information interesting and informative, then share this math article with your friends. If you have come to this website for the first time then follow the website and also follow the email subscription. So that you can get the notification of the new article. If you like the article, share and like it with your friends so that they also benefit. If you have any problems or want to make any suggestions, please comment. Read this article in full.)
Also Read This Article:Uniform convergence
श्रेणी के एकसमान अभिसरण के लिए एमएन परीक्षण (Uniform Convergence By mn test):
- M_{n}-test:Let <f_{n}> be a sequence of functions defined on an interval I.
Let \lim_{n\longrightarrow{\infin}}f_{n}(x)\text{ for all }x\in{I}
Set M_{n}=\text{ sup }\left\{|f_{n}-f(x)|:x\in{I}\right\}
Then <f_{n}> converges uniformly to f if and only if M_{n}\longrightarrow{0}\text{ as } n\rightarrow{\infin}
- उपर्युक्त आर्टिकल में श्रेणी के एकसमान अभिसरण के लिए एमएन परीक्षण (Uniform Convergence By mn test) के बारे में बताया गया है।
| No. | Social Media | Url |
|---|---|---|
| 1. | click here | |
| 2. | you tube | click here |
| 3. | click here | |
| 4. | click here | |
| 5. | Facebook Page | click here |
| 6. | click here |
2 Comments





Just desire to say your article is as astonishing. The clarity for your publish is simply great and i can assume you’re a professional in this
subject. Fine along with your permission allow me to
snatch your feed to stay up to date with impending post. Thank you one million and please
continue the gratifying work.
I am glad that you like my articles. I want to assure you that you will find similar quality articles on this site.