Median in Statistics
1.सांख्यिकी में मध्यका (Median in Statistics),सांख्यिकी में चतुर्थक (Quartiles in Statistics):
सांख्यिकी में मध्यका (Median in Statistics) एक स्थिति-सम्बन्धी माध्य है।यह किसी समंकमाला का वह मूल्य है जो कि समंकमाला को दो भागों में विभाजित करता है।दूसरे शब्दों में,मध्यका आरोही (ascending बढ़ते हुए) अथवा अवरोही (descending गिरते हुए) क्रम में विभिन्न मदों के मध्य (Middle) का मूल्य होता है जिसके ऊपर व नीचे समान संख्या में मूल्य स्थित होते हैं।
आपको यह जानकारी रोचक व ज्ञानवर्धक लगे तो अपने मित्रों के साथ इस गणित के आर्टिकल को शेयर करें।यदि आप इस वेबसाइट पर पहली बार आए हैं तो वेबसाइट को फॉलो करें और ईमेल सब्सक्रिप्शन को भी फॉलो करें।जिससे नए आर्टिकल का नोटिफिकेशन आपको मिल सके । यदि आर्टिकल पसन्द आए तो अपने मित्रों के साथ शेयर और लाईक करें जिससे वे भी लाभ उठाए । आपकी कोई समस्या हो या कोई सुझाव देना चाहते हैं तो कमेंट करके बताएं।इस आर्टिकल को पूरा पढ़ें।
Also Read This Article:-Arithmetic Average in Statistics
2.सांख्यिकी में मध्यका के साधित उदाहरण (Median in Statistics Solved Examples):
Example:20.निम्नलिखित स्थितियों में मध्यका ज्ञात कीजिए:
(Find Median in the following cases):
Example:20(i).एक विद्यार्थी की परीक्षाओं के प्राप्तांक:
84,91,72,87,68,78,95,65
Solution:84,91,72,87,68,78,95,65
आरोही क्रम में रखने पर:
65,68,72,78,84,87,91,95
M=size of \left(\frac{N+1}{2}\right) th item
=size of \left(\frac{8+1}{2}\right) th item
=size of \frac{9}{2} th item
=size of 4.5 th item
=\frac{78+84}{2} \\ \Rightarrow M=81 Marks
Example:20(ii).एक कार्यालय के दस कर्मचारियों का वेतन प्रति घण्टा:
Rs. 2.52,Rs.3.96,Rs.3.28,Rs.9.20,Rs.3.75,Rs.8.65,Rs.7.27,Rs.8.92,Rs.4.25,Rs.6.25
Solution:Rs. 2.52,Rs.3.96,Rs.3.28,Rs.9.20,Rs.3.75,Rs.8.65,Rs.7.27,Rs.8.92,Rs.4.25,Rs.6.25
आरोही क्रम में रखने पर:
2.52,3.28,3.75,3.96,4.25,6.25,7.27,8.65,8.92,9.20
M=size of \left(\frac{N+1}{2}\right) th item
= size of \left(\frac{10+1}{2}\right) th item
= size of \frac{11}{2} th item
= size of 5.5 th item
=\frac{\text { value of } 5 \text { th item }+ \text { value of } 6 \text { th item }}{2} \\ =\frac{4.25+6.25}{2} \\ =\frac{10.50}{2} \\ \Rightarrow M =5.25
Example:20(iii).एक पेड़ के पत्तों की माप (मिलीमीटर में):
119,132,125,135,135,136,138,140,146,138,144,145,145,176,154,149,150,148,173,158
Solution:119,132,125,135,135,136,138,140,146,138,144,145,145,176,154,149,150,148,173,158
आरोही क्रम में रखने पर:
119,125,132,135,135,136,138,138,140,144,145,145,146,148,149,150,154,158,173,176
M=size of \left(\frac{N+1}{2}\right) th item
size of \left(\frac{20+1}{2}\right) th item
=size of \frac{21}{2} th item
= size 10.5 th item
=\frac{\text { value of } 10 \text{ th itam+value of } 11 \text { th item }}{2} \\ =\frac{144+145}{2} \\
\Rightarrow M =144.5 mm
Example:21.एक परीक्षा में 15 विद्यार्थियों के प्राप्तांक निम्नलिखित हैं,मध्यका एवं चतुर्थकों की गणना कीजिए:
(The marks obtained by 15 students in an examination are given below,find out Median and Quartiles):
6,9,10,12,18,19,23,23,24,28,37,48,49,53,60
Solution:6,9,10,12,18,19,23,23,24,28,37,48,49,53,60
आरोही क्रम में रखने पर:
6,9,10,12,18,19,23,23,24,28,37,48,49,53,60
M= size of \left(\frac{(N+1)}{2}\right) th item
=Size of \left(\frac{15+1}{2}\right) th item
= size of 8 th item
M=23
Q_1=size of \left(\frac{N+1}{4}\right) th item
=size of \left(\frac{15+1}{4}\right) th item
=size of 4 th item
\Rightarrow Q_1=12
Q_3=size of 3\left(\frac{N+1}{4}\right) th item
=size of \frac{3(15+1)}{4} thither
=size of 12 th item
Q_3=48
M=23, Q_1=12, Q_3=48
Example:22.निम्न समंकों से मध्यका तथा चतुर्थकों की गणना कीजिए:
(Find median and quartiles of the following data):
Population of cities in thousands:
1486,400,250,182,144,1161,314,214,174,143,647,306,230,160,139,467,275,215,148,137,447,265,205,145,127,430,264,184,144,124
Solution:Population of cities in thousands:
1486,400,250,182,144,1161,314,214,174,143,647,306,230,160,139,467,275,215,148,137,447,265,205,145,127,430,264,184,144,124
आरोही क्रम में रखने पर:
124,127,137,139,143,144,144,145,148,160,174,182,184,205,214,215,230,250,264,265,275,306,314,400,430,447,467,647,1161,1486
M= size of \left(\frac{N+1}{2}\right) th item
=size of \left(\frac{31+1}{2}\right) th item
=size of 15.5 th item
=\frac{\text{ value of 15 th item + value of 16 th item}}{2} \\ =\frac{214+215}{2} \\ \Rightarrow M=214.5 Thousand
Q_1=size of \left(\frac{N+1}{h}\right) th item
= size of \left(\frac{31+1}{4}\right) th item
=size of 7.75 th item
=value of 7 th item + 0.75 (Value of 8th item- Value of 7th item)
=144+0.75(145-144)
=144+0.75 \times 1 \\ \Rightarrow Q_1=144.75 Thousand
Q_3=Size of \frac{3(N+1)}{4} th item
=Size of \frac{3(31+1)}{4} th item
=Size of 23.25 th item
=Size of 23 th item + 0.25(value of 24th item -value of 23th item)
=314+0.25(400-314) \\=314+0.25 \times 86 \\=314+21.5 \\ \Rightarrow Q_3=335.5 Thousand
M=214.5 Thousand, Q_{1}=144.75 Thousand, Q_3=335.5 Thousand
Example:25.एक कक्षा के 12 विद्यार्थियों के तीन विभिन्न प्रश्न-पत्रों में प्राप्तांक निम्नवत हैं।कारण सहित बतलाइए कि किस प्रश्न-पत्र में विद्यार्थियों का बौद्धिक स्तर सर्वोत्तम है:
(12 students of a class obtained the following marks in three different papers.State with reasons, in which paper the level of intelligence is the highest.)
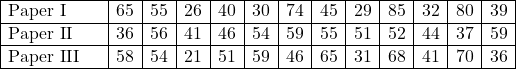
Solution:आरोही क्रम में रखने पर:

Paper I
M=size of \left(\frac{M+1}{2}\right) th item
=size of \left(\frac{12+1}{2}\right) th item
=size of 6.5 th item
= \frac{ \text {value of 6 th item+ value of 7 th item value}}{2}\\ =\frac{40+45}{2} \\ =\frac{85}{2} \\ =42.5 \\ \Rightarrow M=42.5
Paper II
M= \frac{ \text {value of 6 th item+ value of 7 th item value}}{2} \\ M=\frac{51+52}{2}=\frac{103}{2} \\ \Rightarrow M =51.5
M= \frac{ \text {value of 6 th item+ value of 7 th item value}}{2} \\ =\frac{51+54}{2}= \frac{105}{2} \\ \Rightarrow M=52.5
Level is the hightest in paper III
Example:24.निम्नलिखित समंकों से मध्यका तथा चतुर्थकों की गणना कीजिए:
(calculate median and quartiles from the following data):
![]()
Solution:Calculation Table of Median and Quartiles

M=size of \left(\frac{N+1}{2}\right) th item
=size of \left(\frac{115+1}{2}\right) th item
=size of 58 th item M
58वाँ मद देखने के लिए संचयी आवृत्ति देखनी होगी।58 संचयी आवृत्ति 58 में है जिसका पदमूल्य 7 है।अतः M=7
Q_1=size of \left(\frac{N+1}{4}\right) th item
=size of \left(\frac{115+1}{4}\right) th item
= size of 29th item
Q_1=6
Q_3=size of \frac{3(N+1)}{4} th item
= size of \frac{3(115+1)}{4} th item
= size of 87 th item
\Rightarrow Q_3=8
M=7, Q_1=6, Q_3=8 flowers
Example:25.निम्नलिखित सारणी में प्रस्तुत समंकों से मध्यका,प्रथम व तृतीय चतुर्थकों की गणना कीजिए:
(From the data given in the following table,calculate median, quartile first and quartile third.)
![]()
Solution:calculation Table of Median and Quartiles

m=\frac{N}{2}=\frac{100}{2}=50
मध्यका वर्ग 20-30,सूत्रानुसार
M=l_1+\frac{i}{f}(m-c) \\ =20+\frac{10}{40}(50-30) \\=20+\frac{1}{4} \times 20 \\=20+5 \\ \Rightarrow M =25 \\ q_{1}=\frac{N}{4} \\ q_1 =\frac{100}{4}=25
it fall in 10-20 Group
Q_1=l_1+\frac{i}{f}\left(q_{1}-c\right) \\ =10+\frac{10}{20}(25-10) \\ =10+\frac{1}{2} \times 15 \\ =10+7.5 \\ \Rightarrow Q_1 =17.5 \\ q_3=\frac{3 N}{4}=\frac{3 \times 100}{4}=75
it fall in 30-40 Group
Q_3=l_1+\frac{i}{f}\left(q_3-c\right) \\ =30+\frac{10}{15}(75-70) \\ =30+\frac{2}{3} \times 5 \\ =30+\frac{10}{3} \\ =30+3.33 \\ \Rightarrow Q_3 \approx 33.33 marks
M=25, Q_1=17.5, Q_3 \approx 33.3 marks
Example:27.1000 मजदूरों के एक समूह में 4% की मासिक मजदूरी 60 रु. से कम तथा 15% की 62.50 रु. से कम परन्तु 60 रु. से अधिक, 15% की 95 रु. और अधिक परन्तु 100 रु. से कम तथा 5% की 100 रु. और अधिक है।मध्यका मजदूरी तथा निम्न और उच्च चतुर्थक ज्ञात कीजिए।
(In a group of 1000 wage-earners the monthly wages of 4% are below Rs. 60 and that of 15% under Rs. 62.50 but over Rs. 60,15% earned Rs. 95 and over but less than Rs. 100 and 5% got Rs. 100 and over. Find the median wage and the lower and upper quartiles.)
Solution:Calculation Table of Median and Quartiles

m=\frac{N}{2}=\frac{1000}{2}=500
मध्यका वर्ग 62.50-95 है,अतः सूत्रानुसार
M=l_1+\frac{i}{f}\left(m-c\right) \\ =52.50+\frac{32.5}{610}(500-190) \\ =62.50+\frac{32.5 \times 310}{610} \\ =62.50+16.516 \\ =79.016 \\ M \approx 79 \\ q_1=\frac{N}{4}=\frac{1000}{4}=250
It falls in 60.50-95 group
Q_1=l_1+\frac{i}{f}\left(q_1-c\right) \\ =62.50+\frac{32.5}{610}(250-190) \\ =62.50+\frac{32.5 \times 60}{610} \\ =62.50+3.1967 \\ =65.6967
Q_1 \approx 65.70 \\ q_3=\frac{3 N}{4}=\frac{3 \times 1000}{4}= 750
If falls in 60.50-95 group
Q_3=l_1+\frac{i}{f}\left(q_3-c\right) \\ =62.50+\frac{32.5}{610}(750-190) \\ =62.50+\frac{32.5}{610} \times 560 \\ =62.5+\frac{1820}{61} \\=62.5+29.836 \\ =92.336 \\ Q_3 \approx 92.34 \\ M \approx 79, Q_1 \approx 65.70, Q_3 \approx 92.34
उपर्युक्त उदाहरणों के द्वारा सांख्यिकी में मध्यका (Median in Statistics),सांख्यिकी में चतुर्थक (Quartiles in Statistics) को समझ सकते हैं।
3.सांख्यिकी में मध्यका के सवाल (Median in Statistics Questions):
(1.)निम्न समंकों से दोनों चतुर्थकों (both quartiles),चतुर्थ पंचमक (4th quintile),सप्तम अष्टमक (7th octile),नवम दशमक (9th decile) और बारहवाँ शतमक (12th percentile) परिकलित कीजिए:
\begin{array}{|c|c|c|c|c|c|c|c|c|c|} \hline \text{ शब्दों में अक्षरों की संख्या} & 1 & 2 & 3 & 4 & 5 & 6 & 7 & 8 & 9 \\ \hline \text{ शब्दों की संख्या} & 18 & 37 & 41 & 55 & 62 & 48 & 27 & 10 & 1 \\ \hline \end{array}
(2.)अग्र सारणी से चतुर्थकों,तीसरे दशमक (3rd decile) और पैंसठवें शतमक (65th percentile) का परिकलन कीजिए:
\begin{array}{|c|c|}\hline \text{मजदूरी (रु.)} & \text{श्रमिकों की संख्या} \\ \hline 50-59.99 & 11 \\ 50-69.99 & 28 \\ 50-79.99 & 60 \\ 50-89.99 & 68 \\ 50-99.99 & 100 \\ \hline \end{array}
उत्तर (Answers): (1.) Q_1=3, Q_3=6, Q_{n_4}=6, O_7=7, D_9=7, P_{12}=2
(2.) Q_1=68.24, Q_3=85.36, D_3=70.625, P_{65}=81.79
उपर्युक्त सवालों को हल करने पर सांख्यिकी में मध्यका (Median in Statistics),सांख्यिकी में चतुर्थक (Quartiles in Statistics) को ठीक से समझ सकते हैं।
Also Read This Article:-Mean in Statistics
4.सांख्यिकी में मध्यका (Frequently Asked Questions Related to Median in Statistics),सांख्यिकी में चतुर्थक (Quartiles in Statistics) से सम्बन्धित अक्सर पूछे जाने वाले प्रश्न:
प्रश्न:1.मध्यका की परिभाषा दीजिए। (Give the Definition of Median):
उत्तर:डाॅ. ए. एल. बाउले के शब्दों में, “यदि एक समूह के पदों को उनके मूल्यों के आधार पर क्रमबद्ध किया जाये तो लगभग बीच का मूल्य ही मध्यका होता है।” काॅनर के अनुसार, “मध्यका समंक श्रेणी का वह चर मूल्य है जो समूह को दो बराबर भागों में विभाजित करता है,जिसमें एक भाग में सभी मूल्य मध्यका से अधिक और दूसरे भाग में सभी मूल्य उससे कम होते हैं।”
प्रश्न:2.मध्यका की प्रमुख उपयोगिता बताइए। (State the Main Use of Median):
उत्तर:जिन तथ्यों की व्यक्तिगत रूप से पृथक-पृथक तुलना नहीं की जा सकती अथवा जिन्हें समूहों में रखा जाना आवश्यक है,उनकी तुलना के लिए मध्यका प्रयोग बहुत उपयोगी है।इसके द्वारा ऐसी समस्याओं का अध्ययन भी संभव होता है,जिन्हें परिमाण में व्यक्त नहीं किया जा सकता है।उदाहरणार्थ,सुंदरता,बुद्धिमानी,स्वास्थ्य आदि को परिमाण में व्यक्त नहीं कर सकते हैं।इसलिए सामाजिक समस्याओं जैसे मजदूरी,संपत्तियों का विवरण आदि के अध्ययन में मध्यका का प्रयोग विशेष रूप से किया जाता है।व्यावसायिक समस्याओं के अध्ययन में इसका प्रयोग उपयुक्त नहीं होता क्योंकि व्यावसायिक समंक बहुत अस्थिर होते हैं।ऐसी स्थिति में जहां अति-सीमांत मदों को महत्त्व नहीं दिया जाना हो,यह माध्य उपयुक्त रहता है।
प्रश्न:3.मध्यका के दोषों को बताइए। (State Demerits of Median):
उत्तर:(1.)मध्यका की गणना करने के लिए कई बार श्रेणी को आरोही या अवरोही क्रम में व्यवस्थित करना होता है,जो कठिन कार्य होता है।
(2.)यदि मध्यका तथा मदों की संख्या दी गई हो तो भी इनके गुणा करने पर मूल्यों का कुल योग प्राप्त नहीं किया जा सकता है।समांतर माध्य की तरह (\bar{X} \times N=\Sigma X) मध्यका में (M \times N =\Sigma X) नहीं होता है।
(3.)मदों का अनियमित वितरण होने पर मध्यका प्रतिनिधि अंक प्रस्तुत नहीं करता व भ्रमपूर्ण निष्कर्ष निकलते हैं।जैसे एक विद्यार्थी को पांच विषय में क्रमशः 20,10,3,1,0 अंक प्राप्त हुए।इसमें मध्यका अंक 3 प्राप्त हुए जो कि उचित प्रतीत नहीं होते।यह भ्रमपूर्ण निष्कर्ष है।
(4.)जब मदों की संख्या सम (even) है तो मध्यका का सही मूल्य ज्ञात करना संभव नहीं हो पाता।ऐसी स्थिति में मध्यका का मान केवल अनुमानित ही होता है।
(5.)सतत श्रेणी में मध्यका की गणना के लिए आन्तर्गणन का सूत्र प्रयुक्त किया जाता है जिसकी मान्यता है कि वर्ग की समस्त आवृतियां पूरे वर्ग में समान रूप से फैली हुई है जबकि वास्तव में ऐसा न होने पर निष्कर्ष अशुद्ध एवं भ्रामक होते हैं।
(6.)जब बड़े और छोटे मदों को समान भार देना हो तो यह माध्य अनुपयुक्त है क्योंकि यह छोटे-बड़े मदों को छोड़ देता है।
(7.)मध्यका का प्रयोग गणितीय क्रियाओं में नहीं किया जा सकता है।
(8.)मध्यका ज्ञात करते समय,यदि इकाइयों की संख्या में वृद्धि कर दी जावे तो इसका मूल्य बदल जाएगा।
उपर्युक्त प्रश्नों के उत्तर द्वारा सांख्यिकी में मध्यका (Median in Statistics),सांख्यिकी में चतुर्थक (Quartiles in Statistics) के बारे में ओर अधिक जानकारी प्राप्त कर सकते हैं।
| No. | Social Media | Url |
|---|---|---|
| 1. | click here | |
| 2. | you tube | click here |
| 3. | click here | |
| 4. | click here | |
| 5. | Facebook Page | click here |
| 6. | click here |
Median in Statistics
सांख्यिकी में मध्यका
(Median in Statistics)
Median in Statistics
सांख्यिकी में मध्यका (Median in Statistics) एक स्थिति-सम्बन्धी माध्य है।यह किसी समंकमाला
का वह मूल्य है जो कि समंकमाला को दो भागों में विभाजित करता है।दूसरे शब्दों में,मध्यका आरोही
Related Posts
About Author
Satyam
About my self I am owner of Mathematics Satyam website.I am satya narain kumawat from manoharpur district-jaipur (Rajasthan) India pin code-303104.My qualification -B.SC. B.ed. I have read about m.sc. books,psychology,philosophy,spiritual, vedic,religious,yoga,health and different many knowledgeable books.I have about 15 years teaching experience upto M.sc. ,M.com.,English and science.








